Friction
Friction
The following text is used only for teaching, research, scholarship, educational use and informative purpose following the fair use principles.
We thank the authors of the texts and the source web site that give us the opportunity to share their knowledge
Physics
Friction
Friction! You’ve heard that word, heck, maybe you’ve even used it. So what in the world is friction? We've all heard about it and blame it for all sorts of things. It is the cause of many problems, but we couldn't get along without it.
Press your hand down on your desktop and then pull it sideways. The force that tugs on your hand trying to stop it is friction. Friction is experienced by cars rolling down a road, a horse walking in a field, a sailboat gliding through the water, or an airplane cruising at 45,000 feet.
Friction is a force that resists the motion between two objects in contact with one another.
For a fancier definition, try this:
Friction º force caused by interaction of a body with its surroundings.
Cause of Friction:
Friction is brought about by many things. One major cause of friction has to do with the electrons of the two surfaces in contact with one another. When the objects are close, electrons from one object will form weak bonds with the atoms of the other (and vice versa). These bonds, individually weak, are present in great numbers and add up to a significant force. Another cause of friction is the surfaces themselves. Even the smoothest surface is, when examined under high magnification, rough as a cob. When an object lies on a surface, its little hills and dales presses down and gets stuck in the rocky mountains of the other surface. To make them move you have to dislodge all the little catches and hooks that have developed. Once the thing is moving, the surface irregularities still try to catch and lodge with each other.
|
Another cause of friction is deformation of the surface. An object sitting on a surface causes the surface to sag a little. So the object is sort of sitting in a little depression. To make it move, you have to dislodge it from the depression it’s in. (This is similar to what the Physics Kahuna experiences with a class on Monday morning, to get the class moving, he must dislodge it from the depression each student is in.)
Value of Friction: Is friction bad? Yes! Friction is a force that slows things down, makes airplanes go slow, uses up energy, makes you tired, costs a lot of money, wears thing out, &tc.
If friction good? Yes! You couldn't walk without friction. Sans friction, cars wouldn't go and they couldn't be stopped. Your pants would fall off. &tc.
Forces Involved in Friction: Friction opposes motion. If you pull on an object to make it slide across the floor, the frictional force opposes that pull and will be in the opposite direction. The frictional force is always parallel to the surface and in a direction that opposes the motion of the object. There are several forces acting on an object that is experiencing friction.
- The weight of the object which pushes it down onto the surface it sits upon. This is Fg(or mg), the weight vector.
- The force exerted on the object by the surface, pushing it perpendicular to the surface. This is, of course, our old friend, the normal force, n.
- The force that is pulling the object (or pushing it). This is called the applied force, F.
- The frictional force, parallel to the surface and opposing the applied force, f.
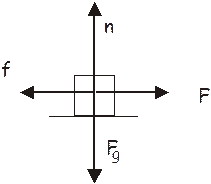
- If the surface is horizontal and there are no other vertical forces n = Fg.
- The normal force, n, is always perpendicular to the surface.
- If F is greater than f, the object must accelerate with a net force of F – f.
- If fis greater than F, the object won't move at all.
- If f = F then the object is moving at a constant speed (or is at rest) as the net force on the object is zero.
Types of Friction: There are several types of friction. The one we'll be dealing with is called sliding friction. There is also rolling friction (which is much smaller). This is the friction of wheels, rollers, ball bearings, &tc. There is also friction caused by passing through fluids - water resistance and air resistance for example.
Figuring Friction: Friction exists between two things. No one, single thing has friction. Friction is the result of the interaction of two surfaces. A rocket traveling through the atmosphere experiences friction with the air but a rocket traveling in the vacuum of space experiences no friction at all. (Well, maybe a teeny amount because the vacuum of space does have a slight smattering of loose atoms and things, but they are so far apart that we can virtually ignore them.)
The magnitude of the frictional force depends on the normal force and the material of the two objects in contact. Wood on wood would have a different frictional force than steel on wood, and so on. Heavy objects have more friction than very light ones, &tc.
When an object is at rest, it takes a larger force to make it move than it takes to keep it moving once it’s got going. Resting objects have to have their static friction overcome before they can move.
Fs º static force of friction
If an object has a force applied to it but remains at rest, then fs = F. The static frictional force can have a max value of F.
Moving objects require an applied force to keep them moving that overcomes the kinetic force of friction. Kinetic is based on a Greek word and means “moving”.
fk º kinetic force of friction.
If the object moves, then the applied force has to be greater than the static frictional force (to get it started) and must be as big or bigger than the kinetic frictional force.
|
|
Kinetic friction is always smaller than static friction.
This is because an object at rest on a surface has its microscopic surfaces embedded in the surface. Also, the electrons form their little bonds (as mentioned before), and you have the depression deal. When an object is in motion, you just have the rough surfaces bouncing off each other. The electrons don’t have time to set up bonds, so that force is near zero, and the depression thing gets eliminated or rendered pretty insignificant. Therefore the kinetic frictional force is always less than the static frictional force.
Finding the frictional force is pretty simple. The frictional force is proportional to the normal force. Since there are two types of friction, static and kinetic, we have two equations:
ms º coefficient of static friction
mk º coefficient of kinetic friction
The value of the coefficients depends on the two surfaces in contact with one another. These values are found by experiment. Useful tables can sometimes be found that have the different coefficient values for common materials worked out and ready for use by the enterprising physicist.
Let’s do a simple friction problem.
- A 25.0 N wood block is pulled across a wooden table at constant speed. What is the force needed to do this? For the coefficient of kinetic friction use 0.35.
The net force must be zero in the x and y direction. This is because the block is not moving up or down, so the sum of the vertical forces must be zero. It is moving at a constant speed horizontally, so , again, the sum of the forces in the x direction must be zero as well.
å Fx = fk – F = 0
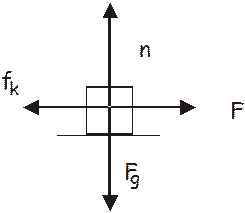so F = fk
Behold! The frictional force is equal to the applied force!
å Fy = n – Fg = 0 therefore n = Fg
Since everything is flat and there are no extra vertical forces, the normal force equals the weight of the block. Knowing the normal force, we can calculate the value of the frictional force, which is, of course, equal to the applied force.
Here is a table of values for some example coefficients of friction.
Let’s look at a slightly more complicated problem where the applied force is at an angle. The force that friction will oppose will be the horizontal component of the applied force.
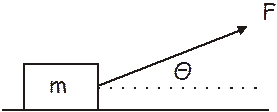You pull a 65.0 kg crate of mass m across the floor with a force F at an angle of 34.0°. The coefficient of friction between the surfaces is 0.235. If the crate moves at a constant speed, what is the applied force?
The complication in this problem is that Fhas a horizontal and vertical component. We draw the FBD. The components are F sin q and F cos q.
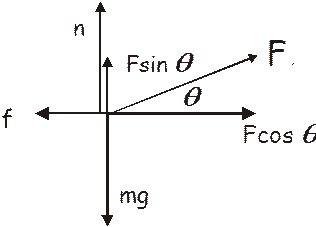
Normally we assume that the normal force which is responsible for the frictional force is equal to the weight of the object, but since there is an upward component from the applied force, this is not true any longer. So we write out an equation for the sum of the forces in the vertical direction and use this equation to find the normal force.
We also know that: 
We now substitute the value for n found in the first equation into the friction equation:
We substitute in the value for the frictional force into the equation for the sum of the horizontal forces and solve the thing for the applied force:
We know what all this stuff is, so we can now plug and chug.
Friction on a Ramp:
Frictional ramp problems are extremely popular with physics affectionados. The basic setup is that a block is on a ramp that is elevated at some angle. The block is at rest, it slides down at a constant speed, it accelerates down the slope, etc. The lucky physics student gets to solve the beast for various things. Let’s look at a simple system. An object rests on the ramp at some angle q.
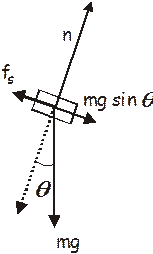
Let us draw a FBD and examine the forces.
The weight of the block is straight down, mg. The normal force, n, is perpendicular to the slope’s surface. There is a component of the weight force directed down the slope, mg sin q. fs is the frictional force which is directed up the ramp.
If q is increased till the block just slides, then fs must equal the force component directed down the ramp.
fs = mg sin q
If block slides down ramp at constant speed, then fk must equal the force component down the ramp
fk = mg sin q
If the block accelerates down the ramp, then the net force down the ramp is:
åFx = mg sin q - fk = ma
Now let’s do a real problem.
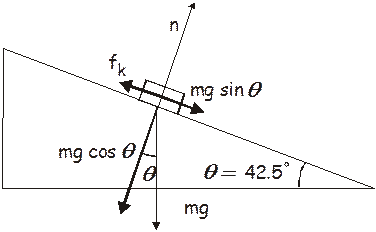
A 15.0 kg block slides down a ramp at constant speed. The elevation angle is 42.5°. What is the coefficient of kinetic friction?
If the block moves at constant velocity, then the net force down the ramp is zero. This means that the kinetic force of friction must equal the force component down the surface of the ramp. First let us find the kinetic force of friction:
å Fx = fk – mg sin q = 0 fk = mg sin q
We know that the force of friction is also: 
Also n = mg cos q so
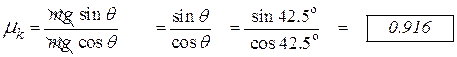
Interesting Point: Look at the solution we got for the coefficient of friction:
From trigonometry we know that: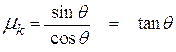 
Thus for constant motion or when the friction force = the force down the slope, the coefficient of friction is simply the tangent of the angle.
Now let’s look at a wonderfully complicated problem. This one is a friction problem crossed with a second law problem like we’ve just mastered.
- A 0.500 kg block is on a ramp attached to a mass hanging over a low friction pulley via a light string. The ramp is elevated at 25.0°. The block is accelerated up the ramp at 0.256 m/s2. What is the hanging mass? For mk use 0.285.
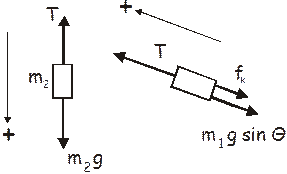First, we draw the appropriate FBD’s. Then we can analyze the forces acting on each block.
We have two equations and two unknowns, T and m2.
We can eliminate T by adding the two equations:
We can now solve this equation for m2: 
But what is fk? Well, we know that it has to be:
So we plug that into the equation we set up for m2:
One could say that this was a complicated problem. To be true, the Physics Kahuna supposes that it probably is. It is not a difficult problem, however. One just has to be methodical – account for all the forces, write out the appropriate equations, and solve for what you need to find. Take your time, be careful, and enjoy the process.
Now we are ready to look at an AP Test problem.
Blocks 1 and 2 of masses m1 and m2, respectively, are connected by a light string, as shown above. These blocks are further connected to a block of mass Mby another light string that passes over a pulley of negligible mass and friction. Blocks 1 and 2 move with a constant velocity v down the inclined plane, which makes an angle q with the horizontal. The kinetic frictional force on block 1 is f and that on block 2 is 2f.
(a) On the figure below, draw and label all the forces on block m1. Express your answers to each of the following in terms of m1, m2, g, q, and f.
- Determine the coefficient of kinetic friction between the inclined plane and block 1.
- Determine the value of the suspended mass Mthat allows blocks 1 and 2 to move with constant velocity down the plane.
(d) The string between blocks 1 and 2 is now cut. Determine the acceleration of block 1 while it is on the inclined plane.
Okay, let’s solve the problem. The first task is simply to draw a FBD. Piece of cake.
(a) On the figure below, draw and label all the forces on block m1.
Express your answers to each of the following in terms of m1, m2, g, q, and f.
We did not draw in the force down the ramp as it is a component of the weight vector, mg which we did draw. It would not have been wrong to draw it in, however. The forces are: the weight, mg; the normal force, n; the tension in the string T1; and the frictional force, f. The frictional force is directed up the ramp because the block is sliding down the ramp and the force of friction is always opposite to the direction the block is moving.
(b) Determine the coefficient of kinetic friction between the inclined plane and block 1.
The coefficient of friction is given by: 
The normal force n is: 
Plug that into the friction equation and we can solve for the coefficient of kinetic friction.
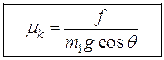
(c) Determine the value of the suspended mass Mthat allows blocks 1 and 2 to move with constant velocity down the plane.
We draw the FBD’s for each of the blocks. We’ve chosen the positive direction to be down the ramp.
å F = 0
For block 1: 
For block 2: 
For block M: 
Add the three equations: 
We do a bunch of algebra stuff:
Solve for M:
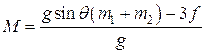
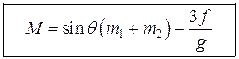
(d) The string between blocks 1 and 2 is now cut. Determine the acceleration of block 1 while it is on the inclined plane.
This is really a simple problem. With the string cut, the tension, T1, is gone and the only forces left are the frictional force, f, and the weight component down the ramp.
å F = m1a 
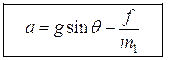
Source : http://teachers2.wcs.edu/high/bhs/mikek/AP%20Physics%20Course%20Notes/Newton%27s%20Laws/3%20-%20friction.doc
Web site link: http://teachers2.wcs.edu
Author : not indicated on the source document of the above text
If you are the author of the text above and you not agree to share your knowledge for teaching, research, scholarship (for fair use as indicated in the United States copyrigh low) please send us an e-mail and we will remove your text quickly.
Friction
Friction
Friction
This is the right place where find the answers to your questions like :
Who ? What ? When ? Where ? Why ? Which ? How ? What does Friction mean ? Which is the meaning of Friction?
Friction physics notes
Alanpedia.com from 1998 year by year new sites and innovations
Main page - Disclaimer - Contact us