Gears
Gears
The following text is used only for teaching, research, scholarship, educational use and informative purpose following the fair use principles.
We thank the authors of the texts and the source web site that give us the opportunity to share their knowledge
Engineering
Gears
Gears:
Introduction: The slip and creep in the belt or rope drives is a common phenomenon, in the transmission of motion or power between two shafts. The effect of slip is to reduce the velocity ratio of the drive. In precision machine, in which a definite velocity ratio is importance (as in watch mechanism, special purpose machines..etc), the only positive drive is by means of gears or toothed wheels.
Figure 4.1
Friction Wheels: Kinematiclly, the motion and power transmitted by gears is equivalent to that transmitted by friction wheels or discs in contact with sufficient friction between them. In order to understand motion transmitted by two toothed wheels, let us consider the two discs placed together as shown in the figure 4.1.
Figure 4.1
When one of the discs is rotated, the other disc will be rotate as long as the tangential force exerted by the driving disc does not exceed the maximum frictional resistance between the two discs. But when the tangential force exceeds the frictional resistance, slipping will take place between the two discs. Thus the friction drive is not positive a drive, beyond certain limit.
Gears are machine elements that transmit motion by means of successively engaging teeth. The gear teeth act like small levers. Gears are highly efficient (nearly 95%) due to primarily rolling contact between the teeth, thus the motion transmitted is considered as positive.
Gears essentially allow positive engagement between teeth so high forces can be transmitted while still undergoing essentially rolling contact. Gears do not depend on friction and do best when friction is minimized.
Some common places that gears can normally be found are:
Printing machinery parts |
Newspaper Industry |
Book binding machines |
Rotary die cutting machines |
Plastics machinery builders |
Injection molding machinery |
Blow molding machinery |
Motorcycle Transmissions (street and race applications) |
Heavy earth moving to personal vehicles |
Agricultural equipment |
Polymer pumps |
High volume water pumps for municipalities |
High volume vacuum pumps |
Turbo boosters for automotive applications |
Marine applications |
Boat out drives |
Special offshore racing drive systems |
Canning and bottling machinery builders |
Hoists and Cranes |
Commercial and Military operations |
Military offroad vehicles |
Automotive prototype and reproduction |
Low volume automotive production |
Stamping presses |
Diesel engine builders |
Special gear box builders |
Many different special machine tool builders |
4.1 Gear Classification: Gears may be classified according to the relative position of the axes of revolution. The axes may be
- Gears for connecting parallel shafts,
- Gears for connecting intersecting shafts,
- Gears for neither parallel nor intersecting shafts.
Gears for connecting parallel shafts
- Spur gears: Spur gears are the most common type of gears. They have straight teeth, and are mounted on parallel shafts. Sometimes, many spur gears are used at once to create very large gear reductions. Each time a gear tooth engages a tooth on the other gear, the teeth collide, and this impact makes a noise. It also increases the stress on the gear teeth. To reduce the noise and stress in the gears, most of the gears in your car are helical.
External contact
Spur gears are the most commonly used gear type. They are characterized by teeth, which are perpendicular to the face of the gear. Spur gears are most commonly available, and are generally the least expensive.
- Limitations: Spur gears generally cannot be used when a direction change between the two shafts is required.
-
Iinternal contact
Advantages: Spur gears are easy to find, inexpensive, and efficient.
Parallel helical gears: The teeth on helical gears are cut at an angle to the face of the gear. When two teeth on a helical gear system engage, the contact starts at one end of the tooth and gradually spreads as the gears rotate, until the two teeth are in full engagement.
Helical gears
(EmersonPower Transmission Corp)Herringbone gears
(or double-helical gears)
This gradual engagement makes helical gears operate much more smoothly and quietly than spur gears. For this reason, helical gears are used in almost all car transmission.
Because of the angle of the teeth on helical gears, they create a thrust load on the gear when they mesh. Devices that use helical gears have bearings that can support this thrust load.
One interesting thing about helical gears is that if the angles of the gear teeth are correct, they can be mounted on perpendicular shafts, adjusting the rotation angle by 90 degrees.
Helical gears to have the following differences from spur gears of the same size:- Tooth strength is greater because the teeth are longer,
- Greater surface contact on the teeth allows a helical gear to carry more load than a spur gear
The longer surface of contact reduces the efficiency of a helical gear relative to a spur gear
Rack and pinion (The rack is like a gear whose axis is at infinity.): Racks are straight gears that are used to convert rotational motion to translational motion by means of a gear mesh. (They are in theory a gear with an infinite pitch diameter). In theory, the torque and angular velocity of the pinion gear are related to the Force and the velocity of the rack by the radius of the pinion gear, as is shown.
Perhaps the most well-known application of a rack is the rack and pinion steering system used on many cars in the past
Gears for connecting intersecting shafts: Bevel gears are useful when the direction of a shaft's rotation needs to be changed. They are usually mounted on shafts that are 90 degrees apart, but can be designed to work at other angles as well.
The teeth on bevel gears can be straight, spiral or hypoid. Straight bevel gear teeth actually have the same problem as straight spur gear teeth, as each tooth engages; it impacts the corresponding tooth all at once.
Just like with spur gears, the solution to this problem is to curve the gear teeth. These spiral teeth engage just like helical teeth: the contact starts at one end of the gear and progressively spreads across the whole tooth.
Straight bevel gears Spiral bevel gears
On straight and spiral bevel gears, the shafts must be perpendicular to each other, but they must also be in the same plane. The hypoid gear, can engage with the axes in different planes.
Hypoid gears (Emerson Power Transmission Corp)
This feature is used in many car differentials. The ring gear of the differential and the input pinion gear are both hypoid. This allows the input pinion to be mounted lower than the axis of the ring gear. Figure shows the input pinion engaging the ring gear of the differential. Since the driveshaft of the car is connected to the input pinion, this also lowers the driveshaft. This means that the driveshaft doesn't pass into the passenger compartment of the car as much, making more room for people and cargo.
Neither parallel nor intersecting shafts : Helical gears may be used to mesh two shafts that are not parallel, although they are still primarily use in parallel shaft applications. A special application in which helical gears are used is a crossed gear mesh, in which the two shafts are perpendicular to each other.
Crossed-helical gears
Worm and worm gear: Worm gears are used when large gear reductions are needed. It is common for worm gears to have reductions of 20:1, and even up to 300:1 or greater.
Many worm gears have an interesting property that no other gear set has: the worm can easily turn the gear, but the gear cannot turn the worm. This is because the angle on the worm is so shallow that when the gear tries to spin it, the friction between the gear and the worm holds the worm in place.
This feature is useful for machines such as conveyor systems, in which the locking feature can act as a brake for the conveyor when the motor is not turning. One other very interesting usage of worm gears is in the Torsen differential, which is used on some high-performance cars and trucks.4.3 Terminology for Spur Gears
Terminology: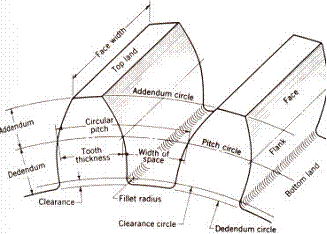
Addendum: The radial distance between the Pitch Circle and the top of the teeth.
Working Depth: The depth to which a tooth extends into the space between teeth on the mating gear.
Arc of Action: Is the arc of the Pitch Circle between the beginning and the end of the engagement of a given pair of teeth.
Arc of Approach: Is the arc of the Pitch Circle between the first point of contact of the gear teeth and the Pitch Point.
Arc of Recession: That arc of the Pitch Circle between the Pitch Point and the last point of contact of the gear teeth.
Backlash: Play between mating teeth.
Base Circle: The circle from which is generated the involute curve upon which the tooth profile is based.
Center Distance: The distance between centers of two gears.
Chordal Addendum: The distance between a chord, passing through the points where the Pitch Circle crosses the tooth profile, and the tooth top.
Chordal Thickness: The thickness of the tooth measured along a chord passing through the points where the Pitch Circle crosses the tooth profile.
Circular Pitch: Millimeter of Pitch Circle circumference per tooth.
Circular Thickness: The thickness of the tooth measured along an arc following the Pitch Circle
Clearance: The distance between the top of a tooth and the bottom of the space into which it fits on the meshing gear.
Contact Ratio: The ratio of the length of the Arc of Action to the Circular Pitch.
Dedendum: The radial distance between the bottom of the tooth to pitch circle.
Diametral Pitch: Teeth per mm of diameter.
Face: The working surface of a gear tooth, located between the pitch diameter and the top of the tooth.
Face Width: The width of the tooth measured parallel to the gear axis.
Flank: The working surface of a gear tooth, located between the pitch diameter and the bottom of the teeth
Gear: The larger of two meshed gears. If both gears are the same size, they are both called "gears".
Land: The top surface of the tooth.
Line of Action: That line along which the point of contact between gear teeth travels, between the first point of contact and the last.
Module: Millimeter of Pitch Diameter to Teeth.
Pinion: The smaller of two meshed gears.
Pitch Circle: The circle, the radius of which is equal to the distance from the center of the gear to the pitch point.
Diametral pitch: Teeth per millimeter of pitch diameter.
Pitch Point: The point of tangency of the pitch circles of two meshing gears, where the Line of Centers crosses the pitch circles.
Pressure Angle: Angle between the Line of Action and a line perpendicular to the Line of Centers.
Profile Shift: An increase in the Outer Diameter and Root Diameter of a gear, introduced to lower the practical tooth number or acheive a non-standard Center Distance.
Ratio: Ratio of the numbers of teeth on mating gears.
Root Circle: The circle that passes through the bottom of the tooth spaces.
Root Diameter: The diameter of the Root Circle.Constant Velocity Ratio
For a constant velocity ratio, the position of P should remain unchanged. In this case, the motion transmission between two gears is equivalent to the motion transmission between two imagined slip-less cylinders with radius R1 and R2 or diameter D1 and D2. We can get two circles whose centers are at O1 and O2, and through pitch point P. These two circles are termed pitch circles. The velocity ratio is equal to the inverse ratio of the diameters of pitch circles. This is the fundamental law of gear-tooth action.
The fundamental law of gear-tooth action may now also be stated as follow (for gears with fixed center distance)
A common normal (the line of action) to the tooth profiles at their point of contact must, in all positions of the contacting teeth, pass through a fixed point on the line-of-centers called the pitch point
Any two curves or profiles engaging each other and satisfying the law of gearing are conjugate curves, and the relative rotation speed of the gears will be constant(constant velocity ratio).
4.2.3 Conjugate Profiles
To obtain the expected velocity ratio of two tooth profiles, the normal line of their profiles must pass through the corresponding pitch point, which is decided by the velocity ratio. The two profiles which satisfy this requirement are called conjugate profiles. Sometimes, we simply termed the tooth profiles which satisfy the fundamental law of gear-tooth action the conjugate profiles.
Although many tooth shapes are possible for which a mating tooth could be designed to satisfy the fundamental law, only two are in general use: the cycloidal and involute profiles. The involute has important advantages; it is easy to manufacture and the center distance between a pair of involute gears can be varied without changing the velocity ratio. Thus close tolerances between shaft locations are not required when using the involute profile. The most commonly used conjugate tooth curve is the involute curve. (Erdman & Sandor).
conjugate action : It is essential for correctly meshing gears, the size of the teeth ( the module ) must be the same for both the gears.
Another requirement - the shape of teeth necessary for the speed ratio to remain constant during an increment of rotation; this behavior of the contacting surfaces (ie. the teeth flanks) is known as conjugate action.4.3 Involute Curve
The following examples are involute spur gears. We use the word involute because the contour of gear teeth curves inward. Gears have many terminologies, parameters and principles. One of the important concepts is the velocity ratio, which is the ratio of the rotary velocity of the driver gear to that of the driven gears.
Generation of the Involute Curve
The curve most commonly used for gear-tooth profiles is the involute of a circle. This involute curve is the path traced by a point on a line as the line rolls without slipping on the circumference of a circle. It may also be defined as a path traced by the end of a string, which is originally wrapped on a circle when the string is unwrapped from the circle. The circle from which the involute is derived is called the base circle.
4.2 Properties of Involute Curves
The line rolls without slipping on the circle.
For any instant, the instantaneous center of the motion of the line is its point of tangent with the circle.
Note: We have not defined the term instantaneous center previously. The instantaneous center or instant center is defined in two ways.
When two bodies have planar relative motion, the instant center is a point on one body about which the other rotates at the instant considered.
When two bodies have planar relative motion, the instant center is the point at which the bodies are relatively at rest at the instant considered.
The normal at any point of an involute is tangent to the base circle. Because of the property (2) of the involute curve, the motion of the point that is tracing the involute is perpendicular to the line at any instant, and hence the curve traced will also be perpendicular to the line at any instant.
The involute profile of gears has important advantages;
- It is easy to manufacture and the center distance between a pair of involute gears can be varied without changing the velocity ratio. Thus close tolerances between shaft locations are not required. The most commonly used conjugate tooth curve is the involute curve. (Erdman & Sandor).
2. In involute gears, the pressure angle, remains constant between the point of tooth engagement and disengagement. It is necessary for smooth running and less wear of gears.
But in cycloidal gears, the pressure angle is maximum at the beginning of engagement, reduces to zero at pitch point, starts increasing and again becomes maximum at the end of engagement. This results in less smooth running of gears.
3. The face and flank of involute teeth are generated by a single curve where as in cycloidal gears, double curves (i.e. epi-cycloid and hypo-cycloid) are required for the face and flank respectively. Thus the involute teeth are easy to manufacture than cycloidal teeth.
In involute system, the basic rack has straight teeth and the same can be cut with simple tools.
Advantages of Cycloidal gear teeth:
1. Since the cycloidal teeth have wider flanks, therefore the cycloidal gears are stronger than the involute gears, for the same pitch. Due to this reason, the cycloidal teeth are preferred specially for cast teeth.
2. In cycloidal gears, the contact takes place between a convex flank and a concave surface, where as in involute gears the convex surfaces are in contact. This condition results in less wear in cycloidal gears as compared to involute gears. However the difference in wear is negligible
3. In cycloidal gears, the interference does not occur at all. Though there are advantages of cycloidal gears but they are outweighed by the greater simplicity and flexibility of the involute gears.
Properties of involute teeth:- A normal drawn to an involute at pitch point is a tangent to the base circle.
2. Pressure angle remains constant during the mesh of an involute gears.
3. The involute tooth form of gears is insensitive to the centre distance and depends only on the dimensions of the base circle.
4. The radius of curvature of an involute is equal to the length of tangent to the base circle.
5. Basic rack for involute tooth profile has straight line form.
6. The common tangent drawn from the pitch point to the base circle of the two involutes is the line of action and also the path of contact of the involutes.
7. When two involutes gears are in mesh and rotating, they exhibit constant angular velocity ratio and is inversely proportional to the size of base circles. (Law of Gearing or conjugate action)
8. Manufacturing of gears is easy due to single curvature of profile.Backlash:
The gap between the non-drive face of the pinion tooth and the adjacent wheel tooth is known as backlash.
If the rotational sense of the pinion were to reverse, then a period of unrestrained pinion motion would take place until the backlash gap closed and contact with the wheel tooth re-established impulsively.Backlash is the error in motion that occurs when gears change direction. The term "backlash" can also be used to refer to the size of the gap, not just the phenomenon it causes; thus, one could speak of a pair of gears as having, for example, "0.1 mm of backlash."
A pair of gears could be designed to have zero backlash, but this would presuppose perfection in manufacturing, uniform thermal expansion characteristics throughout the system, and no lubricant.
Therefore, gear pairs are designed to have some backlash. It is usually provided by reducing the tooth thickness of each gear by half the desired gap distance.
In the case of a large gear and a small pinion, however, the backlash is usually taken entirely off the gear and the pinion is given full sized teeth.
Backlash can also be provided by moving the gears farther apart. For situations, such as instrumentation and control, where precision is important, backlash can be minimised through one of several techniques.References:
- Theory of Machines and Mechanisms by Joseph Edward Shigley and John Joseph Uicker,Jr. McGraw-Hill International Editions.
- Kinematics and Dynamics of Machines by George H.Martin. McGraw-Hill Publications.
- Mechanisms and Dynamics of Machinery by Hamilton H. Mabie and Fred W. Ocvirk. John Wiley and Sons.
- Theory of Machines by V.P.Singh. Dhanpat Rai and Co.
- The Theory of Machines through solved problems by J.S.Rao. New age international publishers.
- A text book of Theory of Machines by Dr.R.K.Bansal. Laxmi Publications (P) Ltd.
- Internet: Many Web based e notes
Gears Trains
A gear train is two or more gear working together by meshing their teeth and turning each other in a system to generate power and speed. It reduces speed and increases torque. To create large gear ratio, gears are connected together to form gear trains. They often consist of multiple gears in the train.
The most common of the gear train is the gear pair connecting parallel shafts. The teeth of this type can be spur, helical or herringbone. The angular velocity is simply the reverse of the tooth ratio.
Any combination of gear wheels employed to transmit motion from one shaft to the other is called a gear train. The meshing of two gears may be idealized as two smooth discs with their edges touching and no slip between them. This ideal diameter is called the Pitch Circle Diameter (PCD) of the gear.
Epicyclic gear train:Epicyclic means one gear revolving upon and around another. The design involves planet and sun gears as one orbits the other like a planet around the sun. Here is a picture of a typical gear box.
This design can produce large gear ratios in a small space and are used on a wide range of applications from marine gearboxes to electric screwdrivers.
Source : http://elearning.vtu.ac.in/syllabus/01%20Power%20Electronics.doc
Web site link: http://elearning.vtu.ac.in/
Google key word : Gears file type : doc
Author : e-Notes by Dr.T.V.Govindaraju, Principal, Shirdi Sai Engineering College, Bangalore
If you are the author of the text above and you not agree to share your knowledge for teaching, research, scholarship (for fair use as indicated in the United States copyrigh low) please send us an e-mail and we will remove your text quickly.
Gears
Gears
Gears
This is the right place where find the answers to your questions like :
Who ? What ? When ? Where ? Why ? Which ? How ? What does Gears mean ? Which is the meaning of Gears?
Gears engineering notes
Alanpedia.com from 1998 year by year new sites and innovations
Main page - Disclaimer - Contact us
